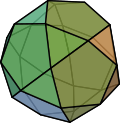
菱形三十面体
 (按这里观看旋转模型) | ||||
| 类别 | 卡塔兰立体 | |||
|---|---|---|---|---|
| 对偶多面体 | 截半二十面体 | |||
| 识别 | ||||
| 鲍尔斯缩写 | rhote | |||
| 数学表示法 | ||||
| 考克斯特符号 | ||||
| 康威表示法 | jD | |||
| 性质 | ||||
| 面 | 30 | |||
| 边 | 60 | |||
| 顶点 | 32 | |||
| 欧拉特征数 | F=30, E=60, V=32 (χ=2) | |||
| 二面角 | 144° | |||
| 组成与布局 | ||||
| 面的种类 | V3.5.3.5 黄金菱形 | |||
| 对称性 | ||||
| 对称群 | Ih, H3, [5,3], (*532) | |||
| 旋转对称群 | Ih, [5,3]+, (532) | |||
| 特性 | ||||
| 凸、等面、等边、环带 | ||||
| 图像 | ||||
| ||||

在几何学中,菱形三十面体(Rhombic triacontahedron)是一个由菱形构成的三十面体[1],由30个全等的黄金菱形组成,具有60条边和32个顶点,其对偶多面体为截半二十面体[2][3]。由于其对偶多面体是一个半正多面体,因此这种立体也属于卡塔兰多面体[4]。
性质
[编辑]菱形三十面体是一个卡塔兰立体[5],由30个面、60条边和32个顶点组成[5],其中30面为12个全等的黄金菱形,因此是一个环带多面体[6]。此外,若将菱形三十面体的边改成与每个面的几何中心相连接[8],则会形成截半二十面体,因此其对偶多面体为截半二十面体[9]。
尺寸
[编辑]若对应的对偶多面体——截半二十面体边长为单位长,则相应的菱形三十面体的体积为[10]:
而相应几何体的边长为[10]:
由此可以推得,如果一个菱形三十面体的棱长为,那么其体积与表面积为[2]:
其中φ为黄金比例。
面的组成
[编辑]组成菱形三十面体的面皆为全等的黄金菱形,其中钝角的角度约为 116.57°,锐角的角度约为 63.43°,两条对角线长度与一边长的比为,长短两对角线长度的比值为黄金比。[5]
分割
[编辑]菱形三十面体可以被分割成20个黄金菱形六面体,包括了10个锐角黄金菱形六面体和10个钝角黄金菱形六面体[12][13]。
| 10 | 10 |
|---|---|
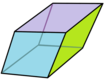 锐角黄金菱形六面体 |
 钝角黄金菱形六面体 |
正交投影
[编辑]菱形三十面体面体有四种具有特殊对称性的正交投影,分别是以面为中心的正交投影、以边为中心的正交投影和两种以顶点为中心的正交投影。其中以度为三的顶点为中心的正交投影应于A2考克斯特平面[14][15];以度为五的顶点为中心的正交投中,其所形成的菱形可以构成潘洛斯镶嵌。[16][17]
| 投影对称性 | [2] | [2] | [6] | [10] |
|---|---|---|---|---|
| 投影位置 | 以面为中心 | 以边为中心 | 度为3的顶点 | 度为5的顶点 |
| 图像 | 
|

|

|

|
| 对偶图像 | 
|
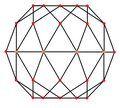
|

|

|
星形化体
[编辑]
菱形三十面体透过全部匹配的星形化方式[18]能够产生227种星形菱形三十面体[19][20]。其中菱形六十面体与五复合立方体为较具代表性的星形菱形三十面体。所有的星形菱形三十面体种类非常繁多,共有358,833,098种星形菱形三十面体,其中包括了84,959个镜像不变的立体和三亿余种具有手性镜像的立体[18]。
其中,菱形六十面体可以透过将菱形三十面体的菱形面沿着长的那一侧向外延长棱直到相交来构造[21]。
-
完全星形菱形三十面体。
用途
[编辑]由于菱形三十面体是一种面可递的立体[22],换句话说,即这立体上的任意两个面A和B,若透过旋转或镜射这个立体,使A移动到B原来的位置时,而两个面仍然占据了相同的空间区域[23]。由于这种特性使得菱形三十面体有时会成为30面骰子的设计[24]。
菱形三十面体亦可用于装饰用途上。丹麦设计师Holger Strøm运用菱形三十面体的结构[25]设计了一种可以手工制作的立体灯饰,称为IQ-light[26],主要以其独特的数学结构形成光影美感,用于制造气氛[27]。亦有艺术家使用菱形三十面体与立方体间的几何关系[2][28]设计出了菱形三十面体造型的收纳盒[29]。
-
三十面骰子。
-
菱形三十面体的灯饰。
菱形三十面体图
[编辑]| 菱形三十面体图 | |
|---|---|
 | |
| 度分布 | 3 (20个) 5 (12个) |
| 顶点 | 32 |
| 边 | 60 |
| 半径 | 6 |
| 直径 | 6 |
| 围长 | 4 |
| 自同构群 | 120 |
| 色数 | 2 |
| 对偶图 | 截半二十面体图 |
| 属性 | 平面图 |
在图论的数学领域中,与菱形三十面体相关的图为菱形三十面体图[11],是菱形三十面体之边与顶点的图,同时也是拓朴结构与菱形三十面体等架的图论对象,由32个节点和60条边组成[30],是一种阿基米德对偶图[31]。尽管菱形三十面体图具备边可递性质,但不具备点可递性质,因此菱形三十面体图不是正则图[32]。
性质
[编辑]菱形三十面体图有60条边和38个顶点,其中度为3的顶点有20个;度为5的顶点有12个[30]。菱形三十面体图不是哈密顿图[30],这意味着菱形三十面体图无法找到一个不重复走访顶点来遍历所有顶点的路径[33]。
 以类似施莱格尔图的方式呈现的菱形三十面体图 |
 菱形三十面体图的另一种表示法 |
参见
[编辑]参考文献
[编辑]- Williams, Robert. The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. 1979. ISBN 0-486-23729-X. (Section 3-9)
- ^ Weisstein, Eric W. (编). Triacontahedron. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ 2.0 2.1 2.2 Weisstein, Eric W. (编). Rhombic Triacontahedron. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Livio Zefiro, DIP.TE.RIS. Review of the alternative choices concerning face colouring of all the regular convex polyhedra and a pair of Catalan polyhedra, the rhombic dodecahedron and the rhombic triacontahedron. mi.sanu.ac.rs. [2020-08-05]. (原始内容存档于2020-07-11).
- ^ The Symmetries of Things 2008, John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, ISBN 978-1-56881-220-5 [1] (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, page 287, pentagonal icosikaitetrahedron)
- ^ 5.0 5.1 5.2 Can, Zeynep and Kaya, Rüstem; et al. On the metrics induced by icosidodecahedron and rhombic triacontahedron. KoG (Hrvatsko društvo za geometriju i grafiku). 2015, 19 (19.): 17–23.
- ^ George W. Hart. Zonohedrification. The Mathematica Journal. 1999, vol. 7 (no. 3) [2018-08-29]. (原始内容存档于2018-11-14).
- ^ Wenninger, Magnus, Dual Models, Cambridge University Press, 1983, ISBN 0-521-54325-8, MR 0730208
- ^ Wenninger (1983)[7], "Basic notions about stellation and duality", p. 1.
- ^ Koca, Mehmet and Koca, Nazife and Koc, Ramazan. Catalan solids derived from three-dimensional-root systems and quaternions. Journal of Mathematical Physics. 2010-04, 51. doi:10.1063/1.3356985.
- ^ 10.0 10.1 Catalan Solids: Rhombic Triacontahedron. dmccooey.com. [2020-08-05]. (原始内容存档于2018-05-08).
- ^ 11.0 11.1 Wolfram, Stephen. "Rhombic triacontahedron". from Wolfram Alpha: Computational Knowledge Engine, Wolfram Research. [7 January 2013] (英语).
- ^ Laszlo C Bardos. Golden Rhombohedra. cutoutfoldup.com. [2020-08-05]. (原始内容存档于2016-04-05).
- ^ George W. Hart. Dissection of the rhombic triacontahedron. Virtual Polyhedra. 1996 [2020-08-05]. (原始内容存档于2021-04-16).
- ^ 约翰·史坦布里奇. Coxeter Planes. math.lsa.umich.edu. (原始内容存档于2018-02-10) (英语).
- ^ 约翰·史坦布里奇. More Coxeter Planes. math.lsa.umich.edu. (原始内容存档于2017-08-21) (英语).
- ^ Kemp, Martin, Science in culture: A trick of the tiles, Nature, 2005, 436 (7049): 332, Bibcode:2005Natur.436..332K, doi:10.1038/436332a
- ^ Livio, Mario, The Golden Ratio: The Story of Phi, the World's Most Astonishing Number, New York: Broadway Books: 206, 2002
- ^ 18.0 18.1 Webb, R. "Enumeration of Stellations.". software3d.com. [2019-09-06]. (原始内容存档于2019-04-27).
- ^ Pawley, G. S. The 227 triacontahedra. Geometriae Dedicata (Kluwer Academic Publishers). 1975, 4 (2–4): 221–232. ISSN 1572-9168. doi:10.1007/BF00148756.
- ^ Messer, P. W. Stellations of the Rhombic Triacontahedron and Beyond. Structural Topology. 1995, 21: 25–46.
- ^ Kabai, Sándor. "Mathematical Graphics I: Lessons in Computer Graphics Using Mathematica.". Püspökladány, Hungary: Uniconstant. 2002: pp. 171, 179, 181.
- ^ Isohedral Rhombohedra. orchidpalms.com. [2020-08-05]. (原始内容存档于2021-04-10).
- ^ McLean, K. Robin, Dungeons, dragons, and dice, The Mathematical Gazette, 1990, 74 (469): 243–256, JSTOR 3619822.
- ^ George W. Hart. Polyhedral Dice. Virtual Polyhedra. 1996 [2020-08-05]. (原始内容存档于2021-04-26).
- ^ Halo Design Group, Geometry, IQ light. halodesign.dk.
- ^ The IQlight concept. halodesign.dk.
- ^ 創客漾思:IQ Light立體燈DIY. 国立频东大学图书馆.[失效链接]
- ^ Weisstein, Eric W. (编). Compound of Five Cubes. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ triacontahedron box - KO Sticks LLC. kosticks.com. [2020-08-05]. (原始内容存档于2021-05-02).
- ^ 30.0 30.1 30.2 30.3 Weisstein, Eric W. (编). Rhombic Triacontahedral Graph. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Weisstein, Eric W. (编). Archimedean Dual Graph. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Weisstein, Eric W. (编). Semisymmetric Graph. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).
- ^ Weisstein, Eric W. (编). Hamiltonian Cycle. at MathWorld--A Wolfram Web Resource. Wolfram Research, Inc. (英语).