克利多胞形
外观
在几何学中,克利多胞形(Kleetope)是多面体的一个类别,是描述一个多面体或更高维度的多胞体,它的面或胞被另一种多面体、锥体替换而产生的几何图形[1]。美国数学家Victor Klee[2]最先描述它们并命名为Kleetope[3],目前其中文名称还没有共识,但命名通常视情况而定,例如在多面体中会议被套用之面之边数命名,如套用于四面体上称为三角化四面体。
例子
[编辑]三角化四面体是四面体经克利变换的像、三角化八面体是八面体经克利变换的像、还有三角化二十面体是二十面体经克利变换的像。在上述每种情况下形成的克利多胞形都是在原始多面体的每个面加入一个三角锥。
 三角化四面体 克利变换的正四面体 |
 四角化六面体 克利变换的立方体 |
 三角化八面体 克利变换的正八面体 |
 五角化十二面体 克利变换的正十二面体 |
 三角化二十面体 克利变换的正二十面体 |
 四角化菱形十二面体 克利变换的菱形十二面体 |
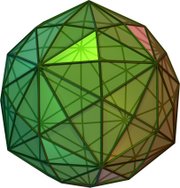 四角化菱形三十面体 克利变换的菱形三十面体 |
 三角化五角化截半二十面体 克利变换的截半二十面体 |
 |
 |
 |
 |
参考文献
[编辑]- Jendro'l, Stanislav; Madaras, Tomáš, Note on an existence of small degree vertices with at most one big degree neighbour in planar graphs, Tatra Mountains Mathematical Publications, 2005, 30: 149–153, MR 2190255.
- Goldner, A.; Harary, F., Note on a smallest nonhamiltonian maximal planar graph, Bull. Malaysian Math. Soc., 1975, 6 (1): 41–42.
- See also the same journal 6(2):33 (1975) and 8:104-106 (1977). Reference from listing of Harary's publications(页面存档备份,存于互联网档案馆).
- Grünbaum, Branko, Unambiguous polyhedral graphs, Israel Journal of Mathematics, 1963, 1 (4): 235–238, MR 0185506, doi:10.1007/BF02759726.
- Grünbaum, Branko, Convex Polytopes, Wiley Interscience, 1967.
- Moon, J. W.; Moser, L., Simple paths on polyhedra, Pacific Journal of Mathematics, 1963, 13: 629–631 [2013-02-14], MR 0154276, (原始内容存档于2016-03-04).
- Plummer, Michael D., Extending matchings in planar graphs IV, Discrete Mathematics, 1992, 109 (1–3): 207–219, MR 1192384, doi:10.1016/0012-365X(92)90292-N.
- ^ Grünbaum (1963, 1967).
- ^ Gritzmann, Peter; Sturmfels, Bernd. Victor L. Klee 1925–2007 (PDF). Notices of the American Mathematical Society (Providence, RI: American Mathematical Society). April 2008, 55 (4): 467–473 [2013-02-14]. ISSN 0002-9920. (原始内容存档 (PDF)于2012-10-10).
- ^ Feature Column from the AMS. American Mathematical Society. [2022-10-15]. (原始内容存档于2022-12-03) (英语).
| 原像 | 截角 | 截半 | 过截角 | 对偶 | 扩展 | 全截 | 交错 | ||
|---|---|---|---|---|---|---|---|---|---|
| 半变换 | 扭棱 | ||||||||

|

|

|

|

|

|

|

| ||
| t0{p,q} {p,q} |
t01{p,q} t{p,q} |
t1{p,q} r{p,q} |
t12{p,q} 2t{p,q} |
t2{p,q} 2r{p,q} |
t02{p,q} rr{p,q} |
t012{p,q} tr{p,q} |
ht0{p,q} h{q,p} |
ht12{p,q} s{q,p} |
ht012{p,q} sr{p,q} |
